Skip to content

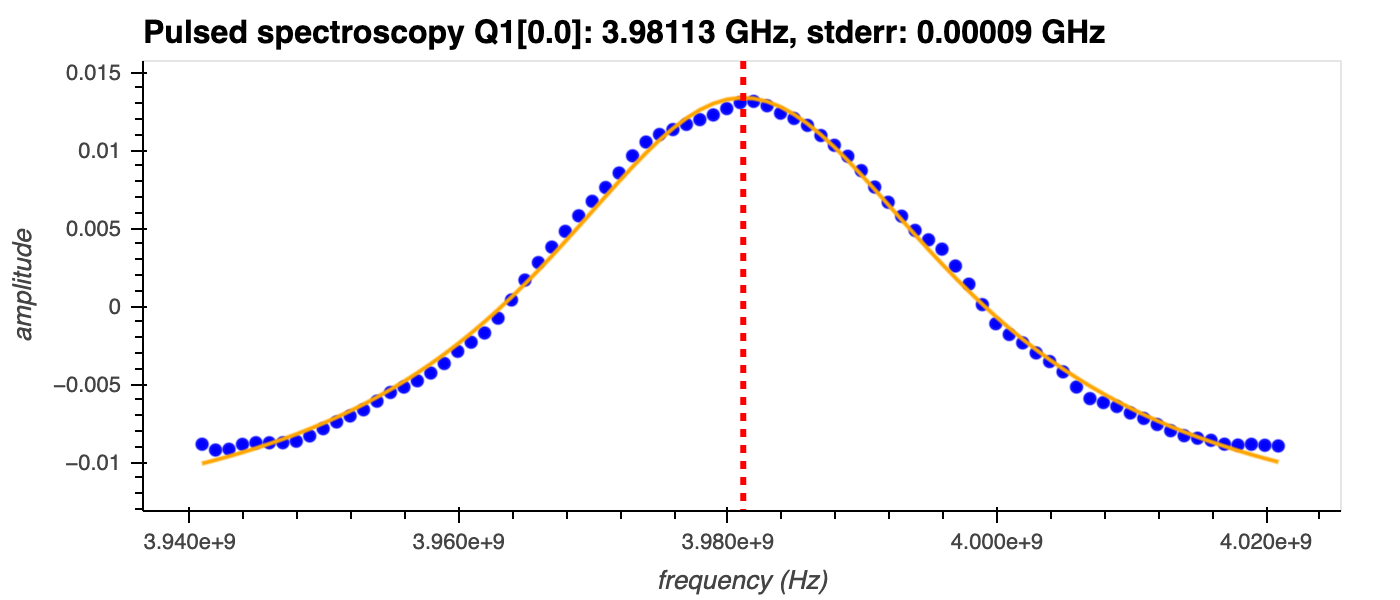
Pulsed qubit spectroscopy

This experiment (also known as two-tone spectroscopy) measures the qubit resonance frequency, \(\omega_q\).

Description

The qubit resonance frequency, \(\omega_q\), is related to the energy difference between the ground (\(E_0\)) and excited states (\(E_1\)) through

\[ E_1-E_0 = \hbar \omega_q , \]

where \(\hbar\) is the reduced Planck's constant.

If frequency of the drive pulse matches \(\omega_q\), the qubit will be (partially) excited; if it does not, it will remain in the ground state. By sweeping the drive frequency around the expected qubit frequency and measuring the resonator response after a (constant) readout pulse is applied, it's possible to determine the value of \(\omega_q\) 1. (Note: the resonator readout frequency must already have been determined. This can be done by performing the Resonator Spectroscopy experiment.)

In superconducting qubits, \(\omega_q\) is usually a few GHz (in the microwave range).

Experiment steps

  1. The qubit drive frequency is swept over a range of values defined around the expected qubit frequency. For each value of the drive frequency, a (constant) readout pulse is applied and the resonator's transmission is measured.

Analysis steps

  1. The amplitude of the resonator's signal is calculated as \(\sqrt{I^2 + Q^2}\), where \(I\) and \(Q\) are the in-phase and quadrature components of the transmitted signal, respectively.

  2. This amplitude is plotted as a function of the drive frequency, and the qubit frequency is extracted from the centre of a Lorentzian shape fit to the data.

image

  1. Marek Pechal. Microwave photonics in superconducting circuits. PhD thesis, ETH Zürich, 2016. doi:10.3929/ethz-a-010735338. ↩