Skip to content

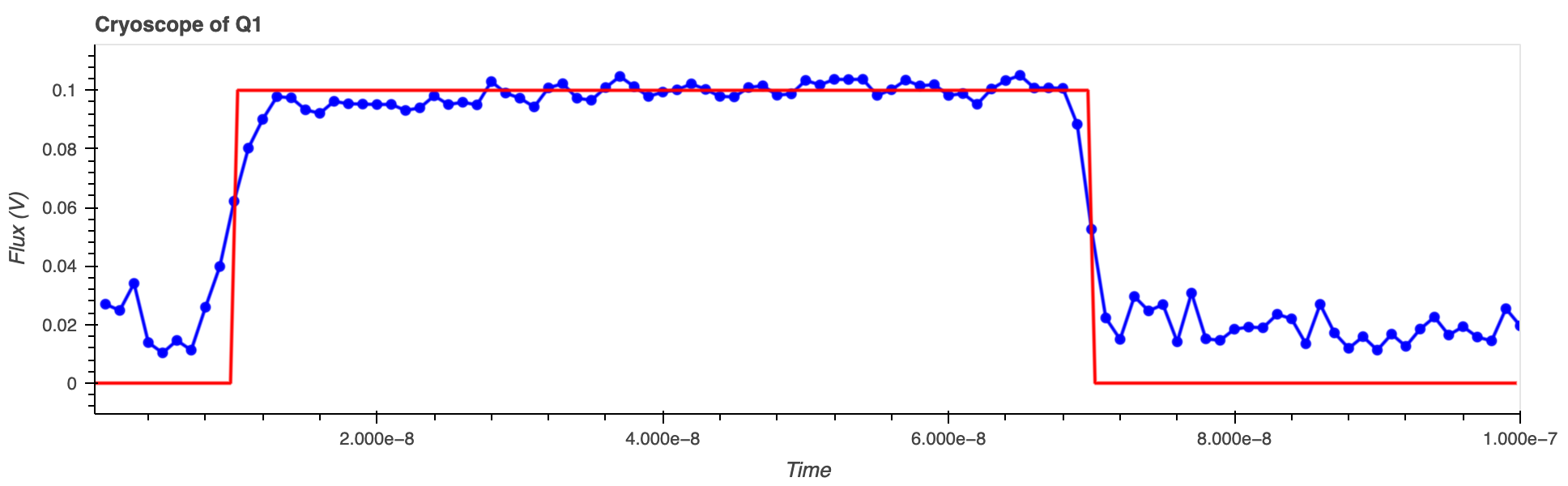
Cryoscope

This experiment reconstructs a flux pulse in the time domain using the methodology described in reference 1.

Description

Achieving high-accuracy dynamical control over qubit frequency is crucial for realising single- and two-qubit gates. In most cases, the control pulse originates from an arbitrary waveform generator (AWG) operating at room temperature. Such a signal experiences dynamical distortions as it passes through electrical components (both inside and outside the dilution refrigerator) on the control line connected to the quantum device, potentially affecting gate performance. While measuring the signal distortion at room temperature is straightforward using, e.g., a Vector Network Analyser; distortions introduced by components inside the refrigerator are temperature-dependent and must be characterised in the cold.

Cryoscope (short for cryogenic oscilloscope) 1 is an in-situ technique that uses the qubit to measure, characterise, and calibrate arbitrarily-shaped control pulses at the same temporal resolution as the AWG. It employs Ramsey-style experiments to obtain an estimate, \(\Phi_{R}(t)\), of the actual flux, \(\Phi(t)\), produced by an AWG pulse \(V_{in}(t)\) as experienced by the qubit. It works for any system with a quadratic or higher power dependence of qubit frequency on the control variable and a sweetspot where qubit frequency is at least first-order insensitive to this variable.

The transition frequency, \(f_{Q}(\Phi)\), of the two-junction transmon is related to the external flux, \(\Phi\), through

\[ f_{Q}(\Phi) \approx \frac{1}{h} \left(\sqrt{8E_{J,\Sigma}E_{C} \left| \cos \left( \pi \frac{\Phi}{\Phi_{0}} \right) \right|} - E_{C}\right), \tag{1} \]

where \(h\) is Planck's constant, \(E_{J,\Sigma}\) is the sum of the Josephson energies of the individual junctions, \(E_{C}\) is the charging energy of the qubit, and \(\Phi_{0}\) is the flux quantum2. Therefore, by measuring the qubit frequency, it's possible to determine the \(\Phi\).

To characterise the temporal behaviour, i.e., \(\Phi(t)\), different segments of the AWG pulse are isolated by truncating (cutting short) the pulse at a time \(\tau\). For instance, if an error is observed after applying a pulse truncated at time \(\tau + \Delta \tau\) that wasn't observed when it was truncated at time \(\tau\), the error must originate in the time interval \(\tau \rightarrow \tau + \Delta \tau\). By varying this time of truncation, it's possible to characterise the full temporal behaviour of the pulse.

\(\frac{\pi}{2}\)-pulse

An initial \(\frac{\pi}{2}\)-pulse prepares the qubit in a superposition state \(\frac{1}{\sqrt{2}}(|0\rangle + |1\rangle)\).

AWG pulse

The truncated AWG pulse generates a magnetic flux \(\Phi_{\tau}(t)\), which induces a phase shift, \(\phi_\tau\), in the qubit's state: \(\frac{1}{\sqrt{2}}(|0\rangle + \text{e}^{\phi_{\tau}}|1\rangle)\).

Third pulse

Two variants of the experiment are performed with a different third pulse for each, one with the final \(\pi/2\) rotation around y and the other with it around x before measuring in order to determine the Bloch vector components \(\langle \sigma_x \rangle\) and \(\langle \sigma_y \rangle\).

\(\langle \sigma_x \rangle\) and \(\langle \sigma_y \rangle\) can then be used to determine the quantum phase, \(\phi_\tau\), accumulated due to the AWG pulse. The derivative of this with respect to \(\tau\), \(\frac{d\phi_\tau}{d\tau}\), is used to calculate the average detuning, \(\overline{\Delta f_{R}}\). In practice, the derivative is estimated by repeating the experiment for small changes \(\Delta \tau\).

\[ \overline{\Delta f_{R}} \equiv \frac{\phi_{\tau + \Delta\tau} - \phi_\tau}{2\pi \Delta\tau}, \tag{2} \]

\(\overline{\Delta f_{R}}\) gives an estimate of \({\Delta f_{Q}}\) up to a quantifiable error \(\varepsilon\) (see equation 4 1). \({\Delta f_{Q}}\) can then be used with Equation 1 to calculate the actual applied flux \(\Phi(t)\).

Experiment steps

  1. \(\frac{\pi}{2}\)-pulse (\(R_x(\frac{\pi}{2})\)) is applied, which prepares the qubit in the superposition state \(\frac{1}{\sqrt{2}}(|0\rangle + |1\rangle)\).

  2. An AWG pulse, \(V_{in,\tau}(t)\), truncated at time \(\tau\) is applied, inducing a phase shift, \(\phi_\tau\), in the qubit's state: \(\frac{1}{\sqrt{2}}(|0\rangle + \text{e}^{\phi_{\tau}}|1\rangle)\).

  3. a. A \(\frac{\pi}{2}\)-pulse (\(R_x(\frac{\pi}{2})\)) is applied to measure the qubit in the \(\sigma_x\)-basis.

    b. A \(\frac{\pi}{2}\)-pulse (\(R_y(\frac{\pi}{2})\)) is applied to measure the qubit in the \(\sigma_y\)-basis.

  4. The resonator transmission is measured.

  5. Steps 1 to 4 are repeated for different values of \(\tau\).

Analysis steps

  1. The discriminator trained in the Readout Discriminator Training experiment is used to determine the qubit state from the \(I\)-\(Q\) values.

  2. The expectation values \(\langle \sigma_x \rangle\) and \(\langle \sigma_y \rangle\) are computed from the measured data in the \(\sigma_x\)- and \(\sigma_y\)-bases, respectively.

  3. The quantum phase, \(\phi_{\tau}\), is computed (\(\phi_{\tau} = \arctan(\langle \sigma_x \rangle, \langle \sigma_y \rangle)\)).

  4. A second-order Savitzky–Golay filter is then used to determine the derivative by fitting a polynomial in a small window around each data point. (\(\phi_{\tau}, \phi_{\tau} + \Delta \tau\)).

  5. The time-dependent reconstructed flux is obtained using Equation 1.

image

  1. M. A. Rol, L. Ciorciaro, F. K. Malinowski, B. M. Tarasinski, R. E. Sagastizabal, C. C. Bultink, Y. Salathe, N. Haandbaek, J. Sedivy, and L. DiCarlo. Time-domain characterization and correction of on-chip distortion of control pulses in a quantum processor. Applied Physics Letters, 116(5):054001, 02 2020. doi:10.1063/1.5133894. ↩↩↩

  2. Jens Koch, Terri M. Yu, Jay Gambetta, A. A. Houck, D. I. Schuster, J. Majer, Alexandre Blais, M. H. Devoret, S. M. Girvin, and R. J. Schoelkopf. Charge-insensitive qubit design derived from the cooper pair box. Phys. Rev. A, 76:042319, Oct 2007. doi:10.1103/PhysRevA.76.042319. ↩