Amplitude Rabi (1-2 states)¶
This experiment measures Rabi oscillations between the first and second excited states of a qubit, and calibrates the amplitude of the \(\pi\)-pulse for this transition.
Description¶
As explained in Amplitude Rabi (0-1 states), if a qubit is driven with a sinusoidal signal with increasing amplitude, one can observe Rabi oscillations as the qubit oscillates between two qubit energy levels. While in the Amplitude Rabi (0-1 states) experiment, these oscillations are between the \(|0\rangle\) and \(|1\rangle\) states, this experiment looks at transitions between the \(|1\rangle\) and \(|2\rangle\) states.
The qubit is first prepared in the \(|1\rangle\) state by applying a \(\pi\)-pulse whose amplitude is determined from Amplitude Rabi (0-1 states). A second drive pulse is then applied to induce a transition to the \(|2\rangle\) state. These two steps are repeated with increasing amplitude of the second drive pulse, leading to Rabi oscillations (see figure below). Since harmonic oscillators do not exhibit this behaviour, Rabi oscillations serve as clear evidence that we are working with qubits, i.e., an anharmonic system1.
This experiment is thus used to determine the amplitude of the \(\pi\)-pulse for the 1-2 transition (\(|1\rangle \rightarrow |2\rangle\)).
Experiment steps¶
-
A \(\pi\)-pulse is applied to prepare the qubit in the first excited state \(|1\rangle\). The amplitude of this pulse should have been previously determined using the Amplitude Rabi (0-1 states) experiment.
-
A second drive pulse with amplitude around that expected for the 1-2 transition is applied to the qubit.
-
The resonator transmission is measured.
-
Steps 1-3 are repeated for different amplitudes of the second drive pulse.
Analysis steps¶
-
The amplitude of the resonator's signal is calculated as \(\sqrt{I^2 + Q^2}\), where \(I\) and \(Q\) are the in-phase and quadrature components of the transmitted signal, respectively.
-
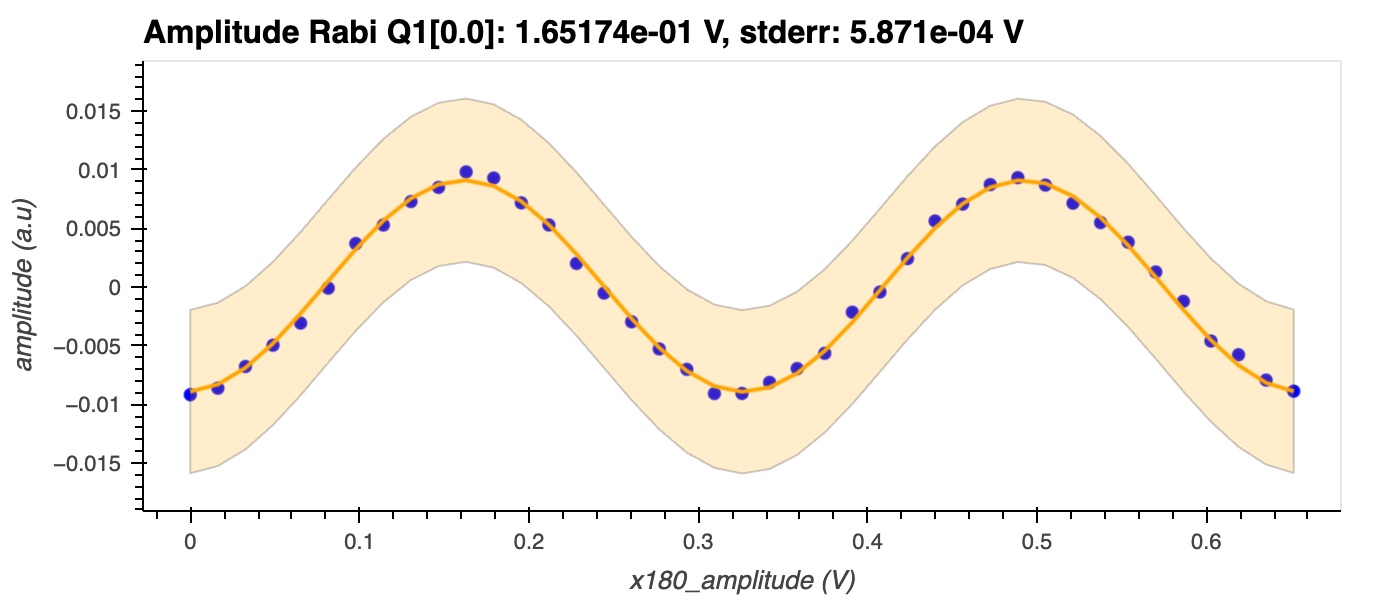
This amplitude is plotted as a function of the amplitude of the second drive pulse, and a sinusoidal function is fit to it. The \(\pi\)-pulse amplitude for the 1-2 transition is determined as the driving pulse amplitude that yields the first maximum of the sinusoid. For example, in the figure below, this occurs at ~0.165.
-
Mahdi Naghiloo. Introduction to experimental quantum measurement with superconducting qubits. 2019. arXiv:1904.09291. ↩